The overestimated quasi-conservative form is implemented to simulate compressible multicomponent flow fields. The WENO scheme is written in the split form that has the consistent and dissipation parts of the numerical flux.

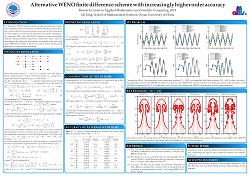
An improved fifth order AWENO-Z scheme has been successfully used for solving nonlinear hyperbolic conservation laws. Here we demonstrate the improved performance of seventh order and ninth order AWENO-Z schemes.

In this paper, the stochastic collocation method and reduced basis method are used to solve the burgers equation, where the reduced basis is obtained by proper orthogonal decomposition(POD).

A fifth order WENO scheme is used to numerically approximate the solution of Euler equation. The performance will be better if the fluxes are projected on the characteristic fields before WENO reconstruction procedure is carried out.

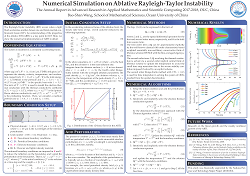
An understanding of the properties of the ARTI, where a low density and high pressure ablating plasma accelerates the high density shell, is a key point for ICF. Here, we show the numerical implementations of ARTI in detail.

An efficient way to represent and track the evolution of interfaces is to use the level-set method. Level set methods add dynamics to implicit surfaces.

Many of the developed high-resolution schemes are based on upwind differencing. The building block of these schemes is the averaging of an approximate Godunov solver. Its time consuming part involves the field-by-field decomposition which is required in order to identify the "direction of the wind".

We design a new modified fifth order WENO-Z scheme.We will give proof of our theory and numerically results on smooth function in the presence of critical points to confirm our theory.