The well-balanced discontinuous Galerkin methods for the shallow water equations can almost maintain the still water steady state exactly, and at the same time can almost preserve the nonnegativity of the water height without loss of mass conservation.

We present a high order numerical scheme for two-dimensional incompressible Navier-Stokes equations based on the vorticity streamfunction formulation with periodic boundary conditions.

我们主要研究了 Multiquadric(MQ) RBF的迭代自适应间断检测方法在一维和二维问题上的应用。对于二维问题，我们采用维数分裂的方法进行处理，这样简单易实现且高效。

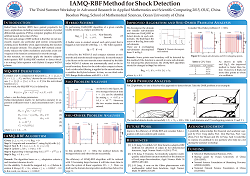
In this research, we apply the iterative adaptive multiquadric RBF (IAMQ-RBF) method to detect the shocks in Hybrid Compact-WENO scheme for solving Euler equations.

In this research, we use IAMQ-RBF method for detection of discontinuities in 1D and 2D problems. For the two-dimensional problems, we use a slice-by-slice approach.

To keep the advantage of high resolution, well-balanced method is used for WENO scheme that we apply same WENO reconstruction on both spatial derivative term and source term.

We discussed a new MPI algorithm so that the MPI program could reduce communication wait time and get the accurate results.

The main objective of this work is to study the 5th order WENO finite difference scheme which is capable of capturing sharp discontinuities in an essentially nonoscillatory manner.

Through the Arakawa C-gird, we simulate the condition of wind-driven circulation, direction of main flow, development of energy, and upper-layer thickness in North Pacific and North Atlantic.

In this work, we investigate the performance of the high order well-balanced Hybrid Compact-WENO scheme for simulations of shallow water equations with source terms due to a non-flat bottom topography.

We mainly show four compact FD schemes (CFDSs), including centered compact scheme, centered compact scheme with spectral-like resolution and two CFDSs for non-uniform grid.

Discontinuous Galerkin (DG) methods are a class of finite element methods using discontinuous basis functions, such as piecewise polynomials. We apply the method to solve the Euler equations with local projection limiting in the characteristic fields.